frachicepcesquidi.netlify.com

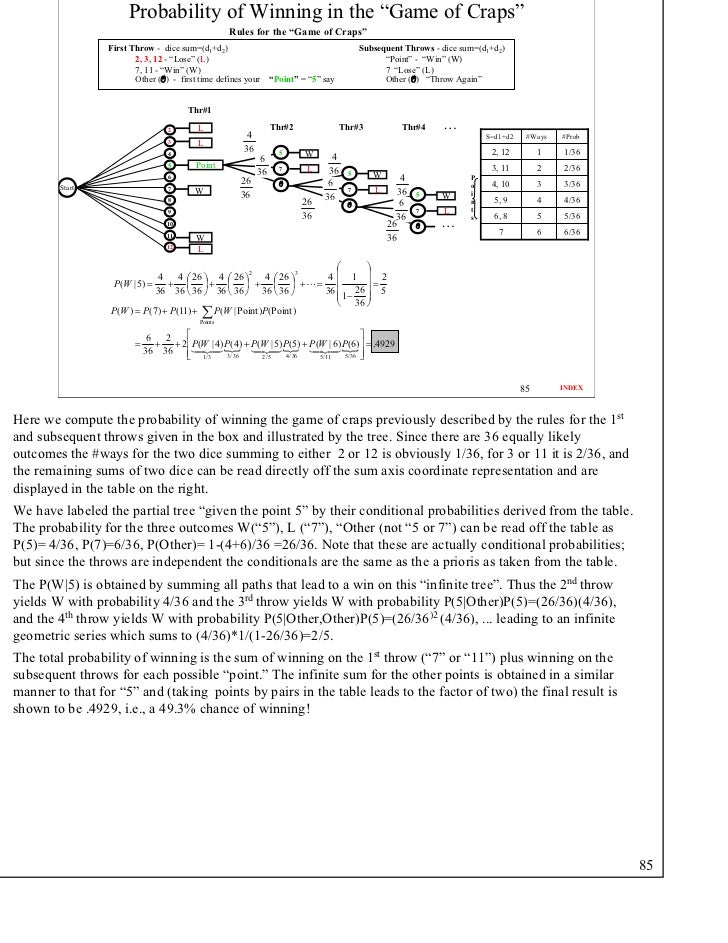
Find the conditional expected number of rolls in the game of craps given that (a)the game does not end on the first roll; (b)the player wins,but not in the first roll. The game of craps is begun by rolling an ordinary pair of dice. If the sum of dice is 2,3 or 12,the player loses. If it is 7 or 11, the player wins. The preceding table shows the probabilities of each roll. (Note that the probability of rolling a 1 with 2 dice is zero; the P1 element is not used for any computations.) You can use the table to compute the probability of winning at craps. If you roll a 7 or 11 on the first roll, you win. If you roll a 2, 3, or 12, you lose. This is not really the probability of making a point. This is the probability of making a point, given that a point is established (ie, it's a conditional probability). The second part is important:) I'd say that, on a come-out roll, the probabilities are: Win with no point (7 or 11): 8 / 36 = 0.22222. This is not really the probability of making a point. This is the probability of making a point, given that a point is established (ie, it's a conditional probability). The second part is important:) I'd say that, on a come-out roll, the probabilities are: Win with no point (7 or 11): 8 / 36 = 0.22222.

1.4-13 Tn the gambling game 'craps' a pair of dice is rolled and the outcome of the experiment is the sum of the points on the up-sides of the six-sided dice. The bettor wins on the first roll if the sum is 7 or 11. The bettor loses on the first roll if the sum is 2, 3, or 12. If the sum is 4, 5, 6, 8, 9, or 10, that number is called the bettor's 'point.' Once the point is established, the rule is, If the bettor rolls a 7 before the 'point,' the bettor loses; hut if the 'point' is rolled before a 7, the bettor wins.
(a) List the 36 outcomes in the sample space for the roll of a pair of dice. Assume that each of them has a probability of 1/36.
(b) Find the probability that the bettor wins on the first roll. That is, fInd the probability of rolling a 7 or ii, P(7 or 11).
(c) Given that 8 is the outcome on the first roll, find the probability that the bettor now rolls the point 8 before rolling a 7 and thus wins. Note that at this stage in the game the only outcomes of interest are 7 and 8. Thus find P(8 7 or 8).
(d) The probability that a bettor rolls an 8 on the first roll and then wins is given by P(8)P(8 7 or 8). Show that this probability is (5/36)(5/11).
(e) Show that the total probability that a bettor wins in the game of craps is 0.49293.
HINT: Note that the bettor can win in one of several mutually exclusive ways: by rolling a 7 or ii on the first roll or by establishing one of the points 4, 5, 6. 8, 9, or 10 on the first roll and then obtaining that point before a 7 on successive rolls.

© BrainMass Inc. brainmass.com October 1, 2020, 8:35 pm ad1c9bdddf
https://brainmass.com/math/probability/conditional-probability-gambling-146405

Attachments

Conditional probability is investigated. The solution is detailed and well presented. The response received a rating of '5/5' from the student who originally posted the question.

Hey Wizard,
I'm a CJ/CIS major working in a Math department, and I've been asked to convert an open ended question test to multiple choice test. I found your site 'The Wizard of Odds', and was directed here to ask my question. What is the probability of rolling a 2 given your roll only being even, when rolling a 20 sided die? Thank you for your help.
-R

I know what you probably meant, but you actually need to specify what's on the 20-sided die. If it's 10 through 200 in increments of 10, the probability is zero.
Under the assumption that the d20 contains integers 1..20, here are the answers I'd list on a four-choice pick:
a) 1/2
b) 1/5
c) 1/10
d) 1/20
Edit: What's a 'CJ' major?

I know what you probably meant, but you actually need to specify what's on the 20-sided die. If it's 10 through 200 in increments of 10, the probability is zero.
Under the assumption that the d20 contains integers 1..20, here are the answers I'd list on a four-choice pick:
a) 1/2
b) 1/5
c) 1/10
d) 1/20
Edit: What's a 'CJ' major?

Funny, I read it differently for the same reason. I 'decided' the question stipulated the 20 sides were numbered 2 thru 20, evens only, with 2 identical sides per number, before I read ME's answer. Be interested to see the clarification.
I'm guessing 'Criminal Justice/Crime Scene Investigation' for the major.

If you're saying 'I've got a d20. The next roll will be an even number. What are the odds of that number being a 2?' Then I'd have to say the odds are 1 in 10. But how does one guarantee that no odd number will be rolled?

It's a conditional probability problem. It's guaranteed because the question says it's guaranteed. It's a math question, not a physics question.Designing multiple-choice tests is one of my favorite things to do. I see this question, and all I think is 'gosh, i wonder how they're doing answer placement. Is it randomized? Rotated? both?'
My HS world geography teacher was a sadistic guy. He put patterns into all his tests, but he also rotated the correct answers. So my 100% score could go a,b,c,d,a,b,c,d... while my friend could have the same answers but need to respond d,c,b,a,d,c,b,a... Made colluding and/or selling answers a real pain.

CJ - Criminal Justice
The numbers on the 20 sided dice are 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20. If this reply sounds smartalically, I appologize. I don't really understand what you mean needing to 'specify what's on the 20-sided die.' Anyhow, which is the answer; a, b, c, or d?

Craps Probabilities

There are 10 even numbers. If you know for sure that it is even, then the answer is 1/10.
You can also solve this by going through the formula for conditional probability. To be honest I always have to look it up (check wikipedia for conditional probability), but you will end up dividing 1/20 by 10/20 and get 1/10.

Craps Conditional Probability Test

Conditional Probability Formula